The Slope Of A Line With Two Points
This topic is about the slope of a line.
Suppose we are given a point \(A\) with the co-ordinate (\(x_1\), \(y_1\)) and a point \(B\) with the co-ordinate \((x_2\), \(y_2\)). The line segment \(AB\) connects the point \(A\) to the point \(B\).
The slope of a line \(m\) represents the ratio of the rise over the run. The rise represents the vertical distance between two points and the run represents the horizontal distance (left to right) between two points.
To compute the slope \(m\) for two points (in the xy-plane), we use the formula:
\[m = \dfrac{y_2 - y_1}{x_2 - x_1}\]
where \(x_2\) is greater than \(x_1\) as we go from left to right in the \(xy\) co-ordinate system.
Here is a visual as a guide:
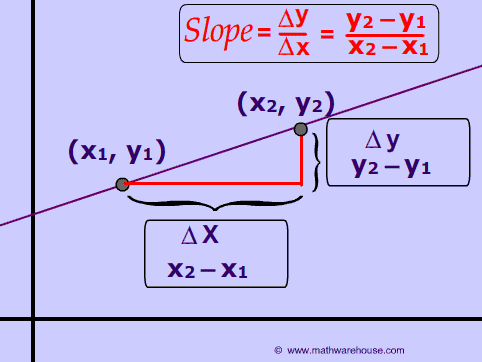
Slope Cases
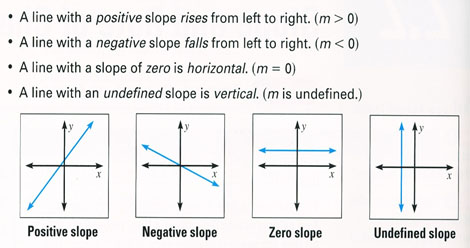
The slope value \(m\) can take on many values but we look at some general cases.
If the value \(m\) is positive or \(m > 0\), we have an upward slope. A \(m\) value which is positive but closer to zero would be a flat upward sloping line. If the \(m\) value is a much larger positive number then the upward sloping line would be steeper and would look closer to a vertical line.
If the value \(m\) is negative or \(m < 0\), we have an downward slope. A \(m\) value which is negative but closer to zero would be a flat downward sloping line. If the \(m\) value is a much larger negative number then the negative sloping line would be steeper and would look closer to a vertical line.
When the \(m\) value is zero, the slope is neither positive nor negative. There is no rise in the run. The line from point \(A\) to point \(B\) is a flat horizontal line.
If \(x_1 = x_2\) then \(x_2 - x_1 = 0\) in the denominator of the slope. A fraction with division by zero is undefined and thus the slope is undefined.
The following picture provides a summary of the cases.
Examples
Example One
Suppose that the point \(A\) is (0, 3) and the point \(B\) is (1, 2). The slope from point \(A\) to point \(B\) is as follows:
\[m = \dfrac{y_2 - y_1}{x_2 - x_1} = \dfrac{2 - 3}{1 - 0} = \dfrac{-1}{1} = -1\]
The slope of the line from \(A\) to \(B\) is downward sloping. For every increase in \(x\) by 1, \(y\) decreases by 1.
Example Two
Given the point \(A\) being (1, 4) and the point \(B\) as (5, 7), the slope from point \(A\) to point \(B\) is:
\[m = \dfrac{y_2 - y_1}{x_2 - x_1} = \dfrac{7 - 4}{5 - 1} = \dfrac{3}{4} \]
The slope of the line from \(A\) to \(B\) is upward sloping. For every increase in \(x\) by 1, \(y\) decreases by \(\dfrac{3}{4}\).